Transfer of Inherent Torsion

Considering two adjacent levels with total storey shear of F_U above and F_L below, the force to be distributed to shear lines on the lower level is F = F_U + F_L which is used to create the direct component of the lower level shear line forces F_di . The torsional component of the forces F_ti depends on the torsion T in the lower diaphragm, which is affected by the torsions and/or forces transmitted by the shear lines from the upper to the lower level.

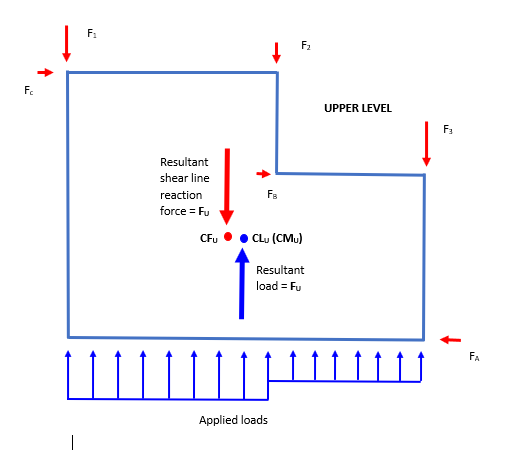
A free body diagram of the upper level diaphragm is shown below. The forces on the upper diaphragm are  

• F_u centered at the center of loading CL_U, which is the center of mass CM_U for seismic design.
• Reactions in the shear lines totaling F_U centered at CF_U. 
• Torsional forces in the shear lines orthogonal to the direction of loading, which sum to zero, but create a torsion counteracting the moment caused by the offset of the forces at CF and CL, which is F_U ( CL_U - CF_U) .

The effect of the shear line forces transferred to the lower level is shown below, where CR_L is the center of rigidity on the lower level.

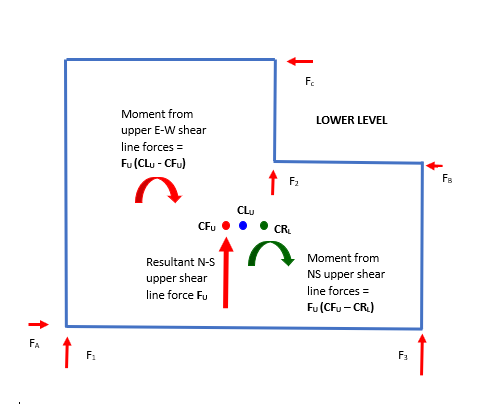
Adding the torsional moments shown, the component T_u coming from the upper level of the torsion T_L on the lower level is

T_U = F_u (CF_U – CR_L)   +  F_U ( CL_U - CF_U) .

= F_u (CL_U – CR_L)

So the model can be simplified by applying the force F_U from the upper level, situated at the center of loading of the upper level (center of mass in seismic design), to the lower level, Thus all the forces acting on the level below can be represented as shown below, in which it is assumed for clarity that there is heavier loading on the left hand side of the lower level than the upper (i.e. due to heavier seismic mass or greater area exposed to wind).

The torsional moment T_L on the lower level is therefore

T_L = F_u (CL_U – CR_L) + F_L (CL_L- - CR_L)

Defining F = F_U + F_L and CL_F as the centroid of the combined upper and lower level force F,

T_L = F_U CL_U + F_L CL_L  +  (F_U + F_L ) CR_L

=   (F_U + F_L )  [ (F_U CL_U   + F_L CL_L ) / (F_U + F_L)  - CR_L  ]

= F ( CL_F +   CR_L  )

So Shearwalls considers the upper level force as a point load at its center of force, and includes it in the torsional analysis of loads on the level below.

Refer to Transfer of Accidental Eccentricity for a continuation of this development that includes accidental eccentricity.